3 april 2017.
Relearning nuclear physics.
Force (in units of 10,000 N) between two nucleons as a function of distance as computed from the Reid potential (1968).[1] The spins of the neutron and proton are aligned, and they are in the S angular momentum state. The attractive (negative) force has a maximum at a distance of about 1 fm with a force of about 25,000 N. Particles much closer than a distance of 0.8 fm experience a large repulsive (positive) force. Particles separated by a distance greater than 1 fm are still attracted (Yukawa potential), but the force falls as an exponential function of distance.
The nuclear force (or nucleon–nucleon interaction or residual strong force) is a force of that acts between protons and neutrons binding them into atomic nuclei.
Neutrons and protons are affected by the nuclear force almost identically.
Since protons have charge +1 e, they experience a strong electric field repulsion (following Coulomb's law) that tends to push them apart, but at short range the attractive nuclear force overcomes the repulsive electromagnetic force.
The mass of a nucleus is less than the sum total of the individual masses of the protons and neutrons which form it.
The difference in mass between a collection of bound nucleons and the same set of unbound nucleons is known as the mass defect and can be expressed as its energy equivalent.
Energy is released when some large nuclei break apart, and it is this energy that is used in nuclear power and nuclear weapons.[2][3]
The nuclear force is powerfully attractive between nucleons at distances of about 1 femtometer (fm, or 1.0 × 10−15 metres), but rapidly decreases to insignificance at distances beyond about 2.5 fm.
At distances less than 0.7 fm, the nuclear force becomes repulsive.
This repulsive component is responsible for the physical size of nuclei, since the nucleons can come no closer than the force allows.
By comparison, the size of an atom, measured in angstroms (Å, or 1.0 × 10−10 m), is five orders of magnitude larger. The nuclear force is not simple, however, since it depends on the nucleon spins, has a tensor component, and may depend on the relative momentum of the nucleons.[4]
A quantitative description of the nuclear force relies on partially empirical equations that model the internucleon potential energies, or potentials. (Generally, forces within a system of particles can be more simply modeled by describing the system's potential energy; the negative gradient of a potential is equal to the vector force.)
The constants for the equations are phenomenological, that is, determined by fitting the equations to experimental data.
The internucleon potentials attempt to describe the properties of nucleon–nucleon interaction. Once determined, any given potential can be used in, e.g., the Schrödinger equation to determine the quantum mechanical properties of the nucleon system.
The discovery of the neutron in 1932 revealed that atomic nuclei were made of protons and neutrons, held together by an attractive force.
By 1935 the nuclear force was conceived to be transmitted by particles called mesons. This theoretical development included a description of the Yukawa potential, an early example of a nuclear potential. Mesons, predicted by theory, were discovered experimentally in 1947.
By the 1970s, the quark model had been developed, by which the mesons and nucleons were viewed as composed of quarks and gluons.
By this new model, the nuclear force, resulting from the exchange of mesons between neighboring nucleons, is a residual effect of the strong force.
Relearning nuclear physics.
Force (in units of 10,000 N) between two nucleons as a function of distance as computed from the Reid potential (1968).[1] The spins of the neutron and proton are aligned, and they are in the S angular momentum state. The attractive (negative) force has a maximum at a distance of about 1 fm with a force of about 25,000 N. Particles much closer than a distance of 0.8 fm experience a large repulsive (positive) force. Particles separated by a distance greater than 1 fm are still attracted (Yukawa potential), but the force falls as an exponential function of distance.
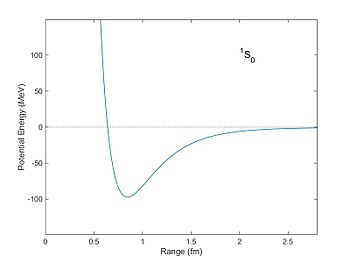
Corresponding potential energy (in units of MeV) of two nucleons as a
function of distance as computed from the Reid potential.
The potential well is a minimum at a distance of about 0.8 fm. With this potential nucleons can become bound with a negative "binding energy."
The potential well is a minimum at a distance of about 0.8 fm. With this potential nucleons can become bound with a negative "binding energy."
Neutrons and protons are affected by the nuclear force almost identically.
Since protons have charge +1 e, they experience a strong electric field repulsion (following Coulomb's law) that tends to push them apart, but at short range the attractive nuclear force overcomes the repulsive electromagnetic force.
The mass of a nucleus is less than the sum total of the individual masses of the protons and neutrons which form it.
The difference in mass between a collection of bound nucleons and the same set of unbound nucleons is known as the mass defect and can be expressed as its energy equivalent.
Energy is released when some large nuclei break apart, and it is this energy that is used in nuclear power and nuclear weapons.[2][3]
The nuclear force is powerfully attractive between nucleons at distances of about 1 femtometer (fm, or 1.0 × 10−15 metres), but rapidly decreases to insignificance at distances beyond about 2.5 fm.
At distances less than 0.7 fm, the nuclear force becomes repulsive.
This repulsive component is responsible for the physical size of nuclei, since the nucleons can come no closer than the force allows.
By comparison, the size of an atom, measured in angstroms (Å, or 1.0 × 10−10 m), is five orders of magnitude larger. The nuclear force is not simple, however, since it depends on the nucleon spins, has a tensor component, and may depend on the relative momentum of the nucleons.[4]
A quantitative description of the nuclear force relies on partially empirical equations that model the internucleon potential energies, or potentials. (Generally, forces within a system of particles can be more simply modeled by describing the system's potential energy; the negative gradient of a potential is equal to the vector force.)
The constants for the equations are phenomenological, that is, determined by fitting the equations to experimental data.
The internucleon potentials attempt to describe the properties of nucleon–nucleon interaction. Once determined, any given potential can be used in, e.g., the Schrödinger equation to determine the quantum mechanical properties of the nucleon system.
The discovery of the neutron in 1932 revealed that atomic nuclei were made of protons and neutrons, held together by an attractive force.
By 1935 the nuclear force was conceived to be transmitted by particles called mesons. This theoretical development included a description of the Yukawa potential, an early example of a nuclear potential. Mesons, predicted by theory, were discovered experimentally in 1947.
By the 1970s, the quark model had been developed, by which the mesons and nucleons were viewed as composed of quarks and gluons.
By this new model, the nuclear force, resulting from the exchange of mesons between neighboring nucleons, is a residual effect of the strong force.
Contents
- 1 Description
- 2 History
- 3 The nuclear force as a residual of the strong force
- 4 Nucleon–nucleon potentials
- 5 See also
- 6 References
- 7 Bibliography
- 8 Further reading
No comments:
Post a Comment